Here you will find a complete list of calculation formulas with decibels divided by topic.
Click the item in the list to start calculating decibels.
- Decibel sum
- Decibel difference
- Calculation of the LAeq equivalent level
- Sound pressure - decibel conversion
- Calculation of sound propagation
- Sound power - sound pressure conversion
- Frequency - wavelength conversion
- Calculation of the level of exposure to noise
DECIBEL SUM
- Insert the LP sound pressure level of any source (in decibel)
- Press the "+" button to add other sources
- Press "sending" or button "=" to calculate the result
 This simple calculation utility, very often sought by those who work in the acoustic sector,
It allows us to notice some peculiarities of physics applied to acoustics.
This simple calculation utility, very often sought by those who work in the acoustic sector,
It allows us to notice some peculiarities of physics applied to acoustics.
First of all, he immediately jumps to the eye that the sum of two sources of equal intensity does not equate absolutely double
of the sound pressure level.
 In reality, the sum of two identical levels involves an increase of only 3 dB, whatever it is
The value in question: (5 db + 5 db = 8 db; 100 dB + 100 dB = 103 db).
In reality, the sum of two identical levels involves an increase of only 3 dB, whatever it is
The value in question: (5 db + 5 db = 8 db; 100 dB + 100 dB = 103 db).
The explanation lies in the fact that when two sources have the same intensity, what in fact doubles is the pressure
sound (P) and not the sound pressure level (LP) . And since the LP level is the logarithm in the base ten in the base
of the pressure P, an additional factor of 3 decibels jumps out for each doubling of the pressure. The formula is the following:
 A further consequence of the fact that the laws of acoustics are governed by logarithms are found when adding
Two lvers of sound pressure, one much higher than the other. The result of the investigation is almost identical to the
greater term. In fact, it is sufficient that the two addends are discouraged by 10 decibels so that the smallest term
become irrelevant for the purpose of the sum.
A further consequence of the fact that the laws of acoustics are governed by logarithms are found when adding
Two lvers of sound pressure, one much higher than the other. The result of the investigation is almost identical to the
greater term. In fact, it is sufficient that the two addends are discouraged by 10 decibels so that the smallest term
become irrelevant for the purpose of the sum.


DECIBEL DIFFERENCE
- Insert the LP sound pressure level of any source (in decibel)
- Press the "-" button to add other sources
- Press "sending" or button "=" to calculate the result
 For the difference of Decibel, the theoretical explanation is the same as above concerning the sum.
For the difference of Decibel, the theoretical explanation is the same as above concerning the sum.
CALCULATION OF THE LAeq EQUIVALENT LEVEL
- Insert the sound LP pressure level of each interval (in decibel)
- Enter the duration of each interval (all in hours or all in minutes ...)
- Press the "+" button to add other contributions
- Press "sending" or button "=" to calculate the result
 This simple calculation utility, very often sought by those who work in the acoustic sector,
It allows us to understand the meaning of the Continuous equivalent level weighted laeq .
This simple calculation utility, very often sought by those who work in the acoustic sector,
It allows us to understand the meaning of the Continuous equivalent level weighted laeq .
The equivalent level is the average integrated over time of the sound pressure level. It therefore defines a unique value
descriptive of the overall noise. Otherwise, however, the sound pressure level is indicative of noise
instant for instant. Therefore the first, as the time of measurement, tends to become constant; the second is constantly
change. In the following figure, which shows the time history of a common phonometric measurement, all this can be
easily displayed. Time History is a graphic designer that represents the trend of the noise level over time. The line
Blue represents the instant sound pressure level, the red line represents the equivalent level.
 Suppose we want to characterize the noise of a certain investigation environment. The noise certainly will not always have
constantly the same intensity. However, we imagine that in this place there are three very noisy main processes
and that follow one another. As seen on the page relating to the sum of sound pressure levels, a much more intense noise than everyone
The others present is equivalent to the overall intensity. Therefore it can be deduced that the overall noise of the environment of
Investigation is given exclusively first by a process, then from the next and finally from the last processing.
If a phonometric detection could not be carried out throughout the working day, some can be measured
minutes of each of the three processing phases. We would get three levels of pressure LP1, LP2, LP3. Knowing the duration T1, T2, T3
processing, by means of the following formula (on which the calculation utility above is based), we will get the same result.
Suppose we want to characterize the noise of a certain investigation environment. The noise certainly will not always have
constantly the same intensity. However, we imagine that in this place there are three very noisy main processes
and that follow one another. As seen on the page relating to the sum of sound pressure levels, a much more intense noise than everyone
The others present is equivalent to the overall intensity. Therefore it can be deduced that the overall noise of the environment of
Investigation is given exclusively first by a process, then from the next and finally from the last processing.
If a phonometric detection could not be carried out throughout the working day, some can be measured
minutes of each of the three processing phases. We would get three levels of pressure LP1, LP2, LP3. Knowing the duration T1, T2, T3
processing, by means of the following formula (on which the calculation utility above is based), we will get the same result.
 A peculiarity of the laws of acoustics, due to the presence of logarithms within the formulas, rediscovers in
fact that individual events of high noise, even if of a reduced duration compared to the rest of the measurement time, become
deciduous for the purposes of the final value of the equivalent level. In a nutshell, an entire size at low noise levels can
Be easily staggered by a single very noisy event, as the equivalent level is significantly affected.
A peculiarity of the laws of acoustics, due to the presence of logarithms within the formulas, rediscovers in
fact that individual events of high noise, even if of a reduced duration compared to the rest of the measurement time, become
deciduous for the purposes of the final value of the equivalent level. In a nutshell, an entire size at low noise levels can
Be easily staggered by a single very noisy event, as the equivalent level is significantly affected.

SOUND PRESSURE - DECIBEL CONVERSION
- Insert the sound pressure P (in micropascal)
- "Send" or button "=" to convert LP (Decibel) sound pressure level
- And viceversa...
Sound pressure → pressure level
pressure level → Sound pressure
 Questa semplice utility di calcolo, molto spesso ricercata da chi opera nel settore dell'acustica,
ci permette di convertire i decibel in pressione e viceversa.
Questa semplice utility di calcolo, molto spesso ricercata da chi opera nel settore dell'acustica,
ci permette di convertire i decibel in pressione e viceversa.
Quando si parla di rumore, si pensa direttamente ai decibel, cioè l'unità di misura dell'intensità di un segnale sonoro.
In realtà però il decibel non è altro che un artificio tecnico per rappresentare l'intera gamma di suoni (dai più forti ai
più deboli) su una scala molto più corta e facilmente memorizzabile. Infatti, il vero indicatore dell'intensità di un suono
è la pressione. Del resto, un'sound wave è essenzialmente una variazione della pressione dell'aria ocomunque del mezzo
elastico entro cui essa si propaga.

A simple example to understand why this conversion artifice: it is much easier to store a plane
It produces 130 dB while the rustle of the 25 dB leaves, instead of memorizing that a plane produces 63.25 PA and the rustle of the
leaves 356 & micro; pa. Furthermore, in this way, the measurement field in which the most common sounds fall to which
we are used to. With a lighter sound, like the rustle of the leaves, to the stronger sound that we can imagine, like a plane
In take -off, on the decibel scale we find only 100 decibels of difference. The totality of the sounds that we can perceive
falls at this interval. If, on the other hand, we reasoned in terms of pressure then we would go from 0.000365 to 63.25 Pascal.
The interval evidently becomes too extensive and difficult to memorize as a order of magnitude. To all this
Obviously the convenience of reasoning is added only with whole numbers.

CALCULATION OF SOUND PROPAGATION
- Insert the level of Sound Pressure known LP (in decibel)
- Insert distance d1 (in metri)
- Insert distance d2 at witch to calc (in metri)
- Press "send" or button "=" to calculate the level of pressure sound (in decibel)
 This simple calculation utility, very often sought by those who work in the acoustic sector,
It allows us to calculate how it propagates the noise is a point source in space.
This simple calculation utility, very often sought by those who work in the acoustic sector,
It allows us to calculate how it propagates the noise is a point source in space.
First of all, it is necessary to explain the difference between pressure level lp and power level lw. The level
of sound power indicates the intrinsic sound of a source and is a unique value. Otherwise the pressure level
sound indicates the sound of a source in the various points of the space for which it depends on the distance. As you move away
From the source the level of pressure sound decreases understandably while the sound power level always remains
The same because it is an objective characteristic of the source.

The conversion from power level to Pressure Level is very useful when you want to predict the noise produced by a specific equipment at a certain distance starting from the data provided by the manufacturer. Usually, in fact The manufacturer provides the power level of the machinery, calculated in the laboratory with special emission tests sound. In the second calculation utility of this page we notice how, for the purposes of conversion from LW to LP, it is necessary The Directivity Factor Q. It depends on the positioning of the source with respect to the support plans. It is easy to guess how The sound emissiveness of a source is much stronger if it is placed in a corner rather than on a horizontal plane. In the first case, in fact, the slice of space through which the noise can be propagated is much smaller therefore the wave of much more concentrated propagation.
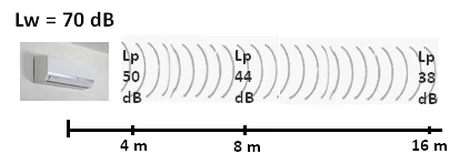
Using the first calculation utility of this page you can easily notice how the pressure level pressure level of one Sound source decreases by 6 decibels at every doubling of the distance. So if an air conditioner emits 66 decibels at 3 meters They will perceive 60 to 6 meters and 54 to 12 meters and so on ...
SOUND POWER - SOUND PRESSION CONVERSION
- Insert the LW sound power (in decibel)
- Insert the directivity Q of the source (see image below)
- Enter the distance D (in meters)
- "Send" or button "=" to convert over the level of pressure sound (in decibel)
- And viceversa...
Sound power → Sound pressure
Sound pressure → Sound power
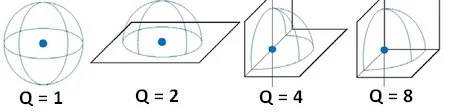
Directivity index of sound propagation
 This simple calculation utility, very often sought by those who work in the acoustic sector,
It allows us to calculate how it propagates the noise is a point source in space.
This simple calculation utility, very often sought by those who work in the acoustic sector,
It allows us to calculate how it propagates the noise is a point source in space.
First of all, it is necessary to explain the difference between pressure level lp and power level lw. The level
of Sound Power indicates the intrinsic sound of a source and is a unique value. Otherwise the pressure level
sound indicates the sound of a source in the various points of the space for which it depends on the distance. As you move away
From the source, the level of Sound pressure decreases understandably while the level of sound power always remains
the same because it is an objective characteristic of the source.

The conversion from power level to Pressure Level is very useful when you want to predict the noise
produced by a specific equipment at a certain distance starting from the data provided by the manufacturer. Usually, in fact
The manufacturer provides the power level of the machinery, calculated in the laboratory with special emission tests
sound. In the second calculation utility of this page we notice how, for the purposes of conversion from LW to LP, it is necessary
The Directivity Factor Q. It depends on the positioning of the source with respect to the support plans. It is easy to guess how
The sound emissiveness of a source is much stronger if it is placed in a corner rather than on a horizontal plane.
In the first case, in fact, the slice of space through which the noise can be propagated is much smaller therefore the wave of
much more concentrated propagation.
Using the first calculation utility of this page you can easily notice how the pressure level pressure level of one
Sound source decreases by 6 decibels at every doubling of the distance. So if an air conditioner emits 66 decibels at 3 meters
They will perceive 60 to 6 meters and 54 to 12 meters and so on ...

FREQUENCY - WAVELENGTH CONVERSION
- Enter the air temperature t (in ° C)
- Enter the sound frequency F (in Hertz)
- Press "sending" or button "=" to convert in wavelength λ (in metri)
- And viceversa...
Frequency → wavelength
wavelength → Frequency
 This simple calculation utility, very often sought by those who work in the acoustic sector,
It allows us to convert the frequency to Wavelength and vice versa. The two sizes, in fact, are strictly
related to each other. They are inversely proportional and their product is equal to the speed of the characteristic sound of the
elastic media of propagation. In the most common case of air, the propagation speed C is equal to 331 meters per second.
With this small calculation it is possible to notice how very high frequencies correspond to very low wavelengths and vice versa.
Therefore it will be easy to remember that a very acute sound (high frequency) has a wave with a large number of close ranges.
On the contrary, a very serious sound (low frequency) has a wave with few and distant oscillations.
This simple calculation utility, very often sought by those who work in the acoustic sector,
It allows us to convert the frequency to Wavelength and vice versa. The two sizes, in fact, are strictly
related to each other. They are inversely proportional and their product is equal to the speed of the characteristic sound of the
elastic media of propagation. In the most common case of air, the propagation speed C is equal to 331 meters per second.
With this small calculation it is possible to notice how very high frequencies correspond to very low wavelengths and vice versa.
Therefore it will be easy to remember that a very acute sound (high frequency) has a wave with a large number of close ranges.
On the contrary, a very serious sound (low frequency) has a wave with few and distant oscillations.

CALCULATION OF THE LEVEL OF EXPOSURE TO NOISE
- Enter the level of Sound pressure LP of each task (in decibel)
- Enter the duration of each task (in minutes)
- Press the "+" button to add other tasks
- Press "sending" or button "=" to calculate the result
 This complex calculation utility, very often sought by those who work in the acoustic sector,
It allows us to calculate, unless the measuring uncertainty, the level of daily noise exposure starting from
Measures relating to each task that composes the job. According to the new UNI 9432: 2011 and 9612: 2011, for each
Three measures must be made in order to define as fully possible as possible the characteristic sound level
of the various tasks. If the three measures differ by over 3 db are not valid for which new phonometric surveys must be made.
The utility does not provide for the calculation of the measuring uncertainty as it depends on numerous factors related to the method of investigation and measurement.
This complex calculation utility, very often sought by those who work in the acoustic sector,
It allows us to calculate, unless the measuring uncertainty, the level of daily noise exposure starting from
Measures relating to each task that composes the job. According to the new UNI 9432: 2011 and 9612: 2011, for each
Three measures must be made in order to define as fully possible as possible the characteristic sound level
of the various tasks. If the three measures differ by over 3 db are not valid for which new phonometric surveys must be made.
The utility does not provide for the calculation of the measuring uncertainty as it depends on numerous factors related to the method of investigation and measurement.
